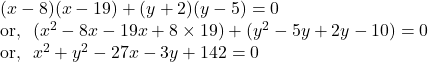In the previous article , we discussed few Short Answer Type Questions. In this article, we will discuss few more Short Answer type Questions from Chhaya Mathematics , Class 11 (S N De book ).

Short Answer Type Questions related to Circle , S N Dey Mathematics | Class 11 | WBCHSE
30. A point moves in such a manner that the sum of the squares of its distance from the origin and the point ![]() is always
is always ![]() Show that the locus of the moving point is a circle. Find the equation to the locus.
Show that the locus of the moving point is a circle. Find the equation to the locus.
Solution.
Suppose that at any instant the co-ordinates of the moving point is ![]()
So, according to the problem,
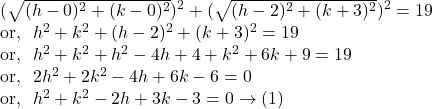
Hence, by ![]() we get the locus of the point
we get the locus of the point ![]() which is
which is ![]() which represents the equation of a circle.
which represents the equation of a circle.
31. ![]() and
and ![]() are two given points and
are two given points and ![]() is a moving point ; if
is a moving point ; if ![]() for all positions of
for all positions of ![]() , show that the locus of
, show that the locus of ![]() is a circle . Find the radius of the circle.
is a circle . Find the radius of the circle.
Solution.
Suppose that at any instant the the co-ordinates of the moving point ![]() is
is ![]()

Hence, by ![]() we get the locus of the point
we get the locus of the point ![]() which is
which is ![]() which represents the equation of a circle.
which represents the equation of a circle.
Comparing the circle with ![]() we get,
we get, ![]()
The radius of the circle is
![]()
32. Show that the locus of the point of intersection of the lines ![]() and
and ![]() when
when ![]() varies, is a straight line.
varies, is a straight line.
Solution.
Suppose that at any instant the point of intersection of two straight lines is ![]()
![]()
From ![]() and
and ![]() we get
we get

So, by ![]() we get the locus of the point of intersection of the lines is
we get the locus of the point of intersection of the lines is
![]() which represents the equation of a circle.
which represents the equation of a circle.
33. Whatever be the values of ![]() , prove that the locus of the point of intersection of the straight lines
, prove that the locus of the point of intersection of the straight lines ![]() and
and ![]() is a circle. Find the equation of the circle.
is a circle. Find the equation of the circle.
Solution.
Suppose that at any instant the point of intersection of two straight lines is ![]()
![]() and
and
![Rendered by QuickLaTeX.com h\sin^3\theta+k\cos^3\theta=a\sin\theta\cos\theta \\ \text{or,}~~ h\sin^3\theta+h\tan\theta\cos^3\theta=a\sin\theta\cos\theta~ ~[\text{By (1)}] \\ \text{or,}~~h\sin^3\theta+h\sin\theta\cos^2\theta=a\sin\theta\cos\theta \\ \text{or,}~~ h\sin\theta(\sin^2\theta+\cos^2\theta)=a\sin\theta\cos\theta \\ \text{or,}~~h\sin\theta=a\sin\theta\cos\theta \\ \text{or,}~~ h=a\cos\theta \rightarrow(2)](https://examhoop.com/wp-content/ql-cache/quicklatex.com-38c8e8daa6632172d61323c1c53d0c2f_l3.png)
Putting the value of ![]() in
in ![]() we get
we get
![]()
Hence, by ![]() we get that the locus of the point of intersection of the straight lines is
we get that the locus of the point of intersection of the straight lines is ![]() which represents the equation of a circle.
which represents the equation of a circle.
34. Show that, ![]() represent a circle passing through the origin. Find the co-ordinates of the centre and length of radius of the circle.
represent a circle passing through the origin. Find the co-ordinates of the centre and length of radius of the circle.
Solution.
![]()
Squaring both sides of ![]() and
and ![]() and adding , we get
and adding , we get
![]()
Clearly, ![]() represents the equation of the circle with centre
represents the equation of the circle with centre ![]() and radius
and radius ![]() unit.
unit.
35. Prove that the square of the distance between the two points ![]() and
and ![]() of the circle
of the circle ![]() is
is ![]()
Solution.
Since the points ![]() and
and ![]() lies on the given circle, so
lies on the given circle, so
![]()
The square of the distance between the two points ![]() and
and ![]() of the circle is
of the circle is
![Rendered by QuickLaTeX.com (\sqrt{(x_1-x_2)^2+(y_1-y_2)^2})^2\\=(x_1-x_2)^2+(y_1-y_2)^2\=x_1^2-2x_1x_2+x_2^2+y_1^2-2y_1y_2+y_2^2\\=(x_1+x_2)^2+(y_1+y_2)^2-2x_1x_2-2y_1y_2\\=a^2+a^2-2x_1x_2-2y_1y_2 ~~[\text{By (1),(2)}]\\=2(a^2-x_1x_2-y_1y_2)~~\text{(proved)}](https://examhoop.com/wp-content/ql-cache/quicklatex.com-75c3316a81a1af61b3635e2695a70812_l3.png)
36. The equations of two diameters of a circle are ![]() and
and ![]() and the length of the chord intercepted on the straight line
and the length of the chord intercepted on the straight line ![]() by the circle is
by the circle is ![]() units. Find the equation of the circle.
units. Find the equation of the circle.
Solution.

The centre of the circle is the intersection of ![]() and
and ![]()
Solving ![]() and
and ![]() , we get
, we get ![]()
So, the centre of the circle is ![]()
From the figure, we notice that ![]()
![]() the distance of the given straight line from the point
the distance of the given straight line from the point ![]()
![]()
From the figure, we notice that the the length of the chord ![]() intercepted on the straight line
intercepted on the straight line ![]() by the circle is
by the circle is ![]() so that
so that ![]()
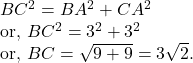
So, the radius of the circle is ![]() unit and centre is
unit and centre is ![]()
![]() the equation of the circle
the equation of the circle

37. Find the equation of the circle circumscribing the rectangle whose sides are given by ![]() and
and ![]()
Solution.

From the figure, we notice that the point ![]() is the intersection of the straight lines
is the intersection of the straight lines![]() and
and ![]()
Now, solving ![]() and
and ![]() we get, the co-ordinates of
we get, the co-ordinates of ![]()
Again, from the figure, we notice that the point ![]() is the intersection of the straight lines
is the intersection of the straight lines ![]() and
and ![]()
So, solving ![]() and
and ![]() we get, the co-ordinates of
we get, the co-ordinates of ![]()
Clearly, ![]() represents the diameter of the circle.
represents the diameter of the circle.
Now, the equation of the diameter ![]() having extremities
having extremities ![]() and
and ![]() is
is