In the previous article , we solved few solutions of Short Answer Type Questions of Ellipse Chapter of S.N.Dey mathematics, Class 11. In this chapter, we will solve few more.

![]() A point moves so that its distance from
A point moves so that its distance from ![]() is
is ![]() times its distance from the line
times its distance from the line ![]() Show that the locus of the moving point is an ellipse whose equation you are to determine.
Show that the locus of the moving point is an ellipse whose equation you are to determine.
Solution.
Let the co-ordinates of any point be ![]() so that distance of the point from the point
so that distance of the point from the point ![]() is
is
![]()
Again, distance of the point ![]() from the line is
from the line is
![]()
By question,
![Rendered by QuickLaTeX.com s=\frac{d}{\sqrt{2}} \\ \text{or,}~~ \sqrt{2}s=d \\ \text{or,}~~ 2s^2=d^2 \\ \text{or,}~~ 2[h^2+(k+3)^2]=\frac{(3h-4k+1)^2}{25} \\ \text{or,}~~ 50(h^2+k^2+6k+9)=(3h-4k+1)^2 \\ \text{or,}~~ 50h^2+50k^2+300k+450=9h^2+16k^2+1+1 \times 3h \times (-4k)+2 \times (-4k) \times 1+2 \times 3h \times 1 \\ \text{or,}~~ 41h^2+34k^2+24hk-6h+308k+449=0.](https://examhoop.com/wp-content/ql-cache/quicklatex.com-31d11608da07007a5f1ba9093a0f30cb_l3.png)
Hence, the equation of the moving point is an ellipse and is given by
![]()
![]() Find the equation of the ellipse whose major axis is parallel to
Find the equation of the ellipse whose major axis is parallel to ![]() axis and
axis and
![]() centre is
centre is ![]() , eccentricity is
, eccentricity is ![]() and the length of latus rectum is
and the length of latus rectum is ![]()
![]() centre is
centre is ![]() length of major axis
length of major axis ![]() and the co-ordinates of foci are
and the co-ordinates of foci are ![]() and
and ![]()
Solution (i)
The length of the latus rectum ![]()
![Rendered by QuickLaTeX.com e=\frac{\sqrt{7}}{4} \Rightarrow e^2=\frac{7}{16} \\ \text{or,}~~1-\frac{b^2}{a^2}=\frac{7}{16} \\ \text{or,}~~ 1-\frac{7}{16}=\frac 1a \cdot \frac{9}{4}~~[\text{By (1)}] \\ \text{or,}~~ \frac{9}{16}=\frac{9}{4a} \\ \text{or,}~~ 4a=16 \\ \text{or,}~~ a=\frac{16}{4}=4.](https://examhoop.com/wp-content/ql-cache/quicklatex.com-1fd039af5da6bd374fe3cfc20e44dae1_l3.png)
![]()
Hence, the equation of the ellipse is given by
![]()
Solution(ii)
![]()
The length of the major axis ![]()
The distance between the foci is
![Rendered by QuickLaTeX.com 2ae=\sqrt{(-1+3)^2+(1-1)^2}=2 \\ \text{or,}~~ ae=1 \\ \text{or,}~~ a^2e^2=1^2 \\ \text{or,}~~ a^2\left(1-\frac{b^2}{a^2}\right)=1 \\ \text{or,}~~ a^2-b^2=1 \\ \text{or,}~~ 3-b^2=1~~[\text{By (1)}] \\ \text{or,}~~ b^2=3-1 \\ \text{or,}~~ b^2=2.](https://examhoop.com/wp-content/ql-cache/quicklatex.com-04d2bb43b5ea23a7ebf33afaec072af1_l3.png)
Hence, the equation of the ellipse is ![]()
![]() Find the equation of the ellipse , for which the foci are
Find the equation of the ellipse , for which the foci are ![]() and
and ![]() and length of the minor axis is
and length of the minor axis is ![]() unit. Explain how the ellipse is reduced to a circle when its two foci coincide.
unit. Explain how the ellipse is reduced to a circle when its two foci coincide.
Solution.
The length of minor axis ![]()
The distance between two foci is given by

From the position of foci we can easily conclude that the ellipse will be of the form

2nd Part :
When two foci coincide, then the distance between two foci ![]()
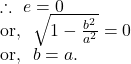
Hence, the equation of the ellipse is reduced into
![]()
So, the equation ![]() represents a circle.
represents a circle.
![]() The eccentricity of an ellipse is
The eccentricity of an ellipse is ![]() focus is
focus is ![]() and the major axis and directrix intersect at
and the major axis and directrix intersect at ![]() Find the co-ordinates of the centre of the ellipse.
Find the co-ordinates of the centre of the ellipse.
Solution.

Let the centre of the ellipse be ![]() Now, the focus
Now, the focus ![]() divides the line segment
divides the line segment ![]() at the ratio
at the ratio ![]() internally.
internally.
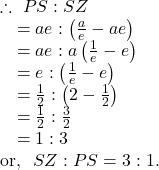
![]()
Hence, the centre of the ellipse is ![]()
![]() The lengths of major and minor axes of an ellipse are
The lengths of major and minor axes of an ellipse are ![]() and
and ![]() and their equations are
and their equations are ![]() and
and ![]() respectively. Find the equation of the ellipse.
respectively. Find the equation of the ellipse.
Solution.
Since the major axis and minor axes are parallel to the ![]() -axis and
-axis and ![]() -axis respectively. So, the equation of the ellipse can be taken in the form
-axis respectively. So, the equation of the ellipse can be taken in the form ![]() where
where ![]() is the centre of the ellipse.
is the centre of the ellipse.
The length of the major axis : ![]()
The length of the major axis : ![]()
Now, the centre of the ellipse is the intersection of ![]() and
and ![]() which is
which is ![]()
Hence, the equation of the ellipse is

![]() Show that the point
Show that the point ![]() lies on the ellipse
lies on the ellipse ![]() Show further that the sum of its distances from two foci is equal to the length of its major axis.
Show further that the sum of its distances from two foci is equal to the length of its major axis.
Solution.

Since the point ![]() satisfies the equation of the ellipse, the point
satisfies the equation of the ellipse, the point ![]() lies on the ellipse.
lies on the ellipse.
Again, ![]()
![]()
So, ![]()
Hence, the co-ordinates of the foci is
![]()
So, the sum of its distances from the two foci is
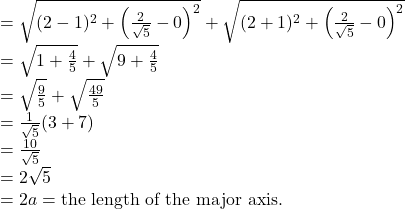
![]() Prove that
Prove that ![]() for the ellipse
for the ellipse ![]() and
and ![]() are two foci of the ellipse of the ellipse and
are two foci of the ellipse of the ellipse and ![]() is any point on the ellipse.
is any point on the ellipse.
Solution.
The equation of the given ellipse is ![]() i.e.,
i.e., ![]()
Comparing ![]() with
with ![]() , we get
, we get ![]()
So, the co-ordinates of any point on the ellipse ![]() can be written as
can be written as ![]()
![]()
So, the co-ordinates of the foci
![]()
