Plane | Part-6 | Ex-5A Examhoop

In the following article, we are going to discuss/solve few Short Answer Type Questions of S.N.Dey Mathematics-Class 12 of the chapter Plane. In the previous article , we have solved few Short Answer type Questions.

10. If the points $~(2-x, 2, 2), ~(2, 2-y, 2)~$ and $~(2, 2, 2-z)~$ are coplanar then prove that $~\frac 2x+\frac 2y+\frac 2z=1.$

Solution.

If the points $~(2-x,2,2),~(2,2-y,2)~$ and $~(2,2,2-z)~$ are coplanar then

$\begin{vmatrix} 2-x& 2 & 2 \\ 2& 2-y & 2 \\ 2& 2 &2-z \\ \end{vmatrix}=0 \\ \text{or,}~~\begin{vmatrix} -x & 0 &z \\ 0& -y &z \\ 2& 2 &2-z \\ \end{vmatrix}=0~~[\text{By}~~R_1' \rightarrow R_1-R_3,~R_2' \rightarrow R_2-R_3] \\ \text{or,}~~ -x\begin{vmatrix} -y& z \\ 2& 2-z \\ \end{vmatrix}+z\begin{vmatrix} 0 & -y \\ 2& 2 \\ \end{vmatrix} =0 \\ \text{or,}~~-x[-y(2-z)-2z]+z[0+2y]=0 \\ \text{or,}~~ xy(2-z)+2zx+2yz=0 \\ \text{or,}~~ 2xy+2yz+2zx=xyz \\ \text{or,}~~\frac{2xy+2yz+2zx}{xyz}=1 \\ \therefore~~\frac 2x+\frac 2y+\frac 2z=1~~\text{(proved)}$

11. Let $~\vec{n}~$ be a vector of magnitude $~2\sqrt{3}~$ such that it makes equal acute angles with the coordinate axes. Find the vector and cartesian forms of the equation of a plane passing through $~(1, -1, 2)~$ and normal to $~\vec{n}$ .

Solution.

Suppose that the vector $~\vec{n}~$ makes an angle $~\theta~(\leq 90^{\circ})~$ with the coordinate axes.

$\therefore~ \vec{n}=2\sqrt{3}(\hat{i}\cos\theta+\hat{j}\cos\theta+\hat{k}\cos\theta)$

$\text{Again,}~\cos^2\theta+\cos^2\theta+\cos^2\theta=1 \\ \text{or,}~~ 3\cos^2\theta=1 \Rightarrow \cos\theta=\frac{1}{\sqrt{3}}~~(\because~\theta \leq 90^{\circ})$

$\therefore~~\vec{n}=2\sqrt{3} \left(\frac{\hat{i}}{\sqrt{3}}+\frac{\hat{j}}{\sqrt{3}}+\frac{\hat{k}}{\sqrt{3}}\right) \\ \text{or,}~~\vec{n}=2\hat{i}+2\hat{j}+2\hat{k}.$

So, the equation of the plane passing through $~(1,-1,2)~$ and normal to $~\vec{n}~$ is given by

$~\vec{n} \cdot [\vec{r}-(\hat{i}-\hat{j}+2\hat{k})]=0 \\ \text{or,}~~ (2\hat{i}+2\hat{j}+2\hat{k}) \cdot [\vec{r}-(\hat{i}-\hat{j}+2\hat{k})]=0 \\ \text{or,}~~ (2\hat{i}+2\hat{j}+2\hat{k})\cdot \vec{r}-(2\hat{i}+2\hat{j}+2\hat{k}) \cdot (\hat{i}-\hat{j}+2\hat{k})=0 \\ \text{or,}~~ 2\vec{r} \cdot (\hat{i}+\hat{j}+\hat{k})-(2-2+4)=0 \\ \text{or,}~~2\vec{r} \cdot (\hat{i}+\hat{j}+\hat{k})-4=0 \\ \text{or,}~~ \vec{r} \cdot (\hat{i}+\hat{j}+\hat{k})=2 \rightarrow(1)$

So, the equation $~(1)~$ represents the vector equation of the plane. Now, putting $~\vec{r}=x\hat{i}+y\hat{j}+z\hat{k}~$ in (1) we get,

$~(x\hat{i}+y\hat{j}+z\hat{k}) \cdot (\hat{i}+\hat{j}+\hat{k})=2 \Rightarrow~~ x+y+z=2.$

$\therefore~~ x+y+z=2~~$ represents the cartesian equation of the plane.

12. Through the point $~P(\alpha,\beta,\gamma)~$ a plane is drawn perpendicular to $~OP~$ where $~O~$ is the origin. Let this plane meet the coordinate axes at $~L,~M~$ and $~N.~$ Show that the area of the triangle $~LMN=\frac{r^5}{(2\alpha \beta \gamma)}~$ where $~|\vec{OP}|=r.$

Solution.

The direction ratios of $~\vec{OP}~$ are $~ \alpha-0,~\beta-0,~\gamma-0~$ or, $~\alpha,\beta,\gamma.$

$\therefore~|\vec{OP}|=r \Rightarrow \sqrt{\alpha^2+\beta^2+\gamma^2}=r \rightarrow(1)$

Again, $~OP~$ is normal to the plane . The equation of the plane through the point $~(\alpha,\beta,\gamma)~$ and perpendicular to the plane is

$~\alpha(x-\alpha)+\beta(y-\beta)+\gamma(z-\gamma)=0 \\ \text{or,}~~ \alpha x-\alpha^2+\beta y-\beta^2+\gamma z-\gamma^2=0 \\ \text{or,}~~ \alpha x+\beta y+\gamma z=\alpha^2+\beta^2+\gamma^2 \\ \text{or,}~~ \alpha x+\beta y+\gamma z=r^2~[\text{By (1)}] \\ \therefore~~ \frac{x}{r^2/\alpha}+\frac{y}{r^2/\beta}+\frac{z}{r^2/\gamma}=1 \\ \therefore~~ L \equiv \left(\frac{r^2}{\alpha},0,0\right),~~ M \equiv \left(0,\frac{r^2}{\beta},0\right),~N \equiv \left(0,0,\frac{r^2}{\gamma}\right).$

So, the direction ratios of $~OL, ~OM~$ and $~ON~$ are $~~\frac{r^2}{\alpha},0,0;~~0,\frac{r^2}{\beta},0~~\text{and}~~0,0,\frac{r^2}{\gamma}~$ respectively.

The Volume of $~OLMN$

$\frac 16\begin{vmatrix} \frac{r^2}{\alpha}&0 &0 \\ 0& \frac{r^2}{\beta} &0 \\ 0& 0 &\frac{r^2}{\gamma} \\ \end{vmatrix}=\frac 16\cdot \frac{r^2}{\alpha} \cdot \frac{r^2}{\beta} \cdot \frac{r^2}{\gamma}=\frac{r^6}{6\alpha \beta \gamma}$

Again, the volume of $~~OLMN=\frac 13 \times OP \times \Delta LMN$

$\therefore~ \frac{r^6}{6\alpha \beta \gamma}=\frac 13 \times r \times \Delta LMN \\ \text{or,}~~ \Delta LMN=\frac{r^5}{2\alpha \beta \gamma}~~(\text{proved})$

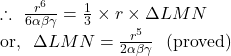
13. A variable plane which is at a constant distance $~3p~$ from the origin $~O~$ cuts the axes at $~L,~M~$ and $~N.~$ Show that the locus of the point of intersection of the planes through $~L,~M,~N~$ drawn parallel to the coordinate axes parallel to the coordinate planes is $~9(x^{-2}+y^{-2}+z^{-2})=p^{-2}.$

Solution.

Let $~P(\alpha,\beta,\gamma)~$ be the point of intersection of three planes passing through the points $~L,~M~$ and $~N.$

The equation of planes passing through $~P~$ and parallel to $~xy,~yz~$ and $~zx~$ planes are $~z=\gamma,~x=\alpha~$ and $~y=\beta~$ respectively and these planes meet z-axis, x-axis and y-axis at $~N(0,0,\gamma),~L(\alpha,0,0)~$ and $~M(0,\beta,0)~$ respectively.

The equation of the plane through the points $~L,M,N~$ can be written as

$~\frac{x}{\alpha}+\frac y\beta +\frac z\gamma=1 \longrightarrow(1)$

Now, the distance of the plane (1) from the origin is $~3p~$ unit.

$\therefore~ \frac{\left|\frac 0\alpha+\frac 0\beta+\frac 0\gamma -1\right|}{\sqrt{\frac{1}{\alpha^2}+\frac{1}{\beta^2}+\frac{1}{\gamma^2}}}=3p \\ \text{or,}~~ 3p \sqrt{\frac{1}{\alpha^2}+\frac{1}{\beta^2}+\frac{1}{\gamma^2}}=1 \\ \text{or,}~~ 9p^2\left(\frac{1}{\alpha^2}+\frac{1}{\beta^2}+\frac{1}{\gamma^2}\right)=1 \\ \text{or,}~~ 9(\alpha^{-2}+\beta^{-2}+\gamma^{-2})=p^{-2}.$

Hence, the locus of the point $~P(\alpha,\beta,\gamma)~$ is $~9(x^{-2}+y^{-2}+z^{-2})=p^{-2}~~(\text{proved})$

14. A variable plane passes through the point $~(f,g,h)~$ and meets the axes at $~L,M~$ and $~N.$ If the planes through $~L,M,N~$ and parallel to the axes meet at $~P~$ , then prove that the locus of $~P~$ is $~\frac fx+\frac gy+\frac hz=1.$

Solution.

Let the equation of the plane be $~\frac x\alpha+\frac y\beta+\frac z\gamma=1.~$ If this plane passes through $~(f,g,h),~$ then

$~\frac f\alpha+\frac g\beta+ \frac h\gamma=1 \longrightarrow(1)$

$\therefore~ L \equiv (\alpha,0,0),~~M \equiv (0,\beta,0),~~N \equiv (0,0,\gamma).$

The equation of the planes through $~L,M,N~$ are $~ x=\alpha,~y=\beta,~ z=\gamma~$ respectively.

So, using (1) we get, $~\frac fx+\frac hy+\frac hz=1\rightarrow(2)$

So, from (2) we get the locus of P and hence the result follows. (proved)

15. A point moves on the plane $~\frac xa+\frac yb+\frac zc=1.~$ The plane, drawn through perpendicular to $~OP~$ meets the axes in $~L,M,N.~$ If the planes through $~L,M,N,~$ parallel to the coordinate planes meet in a point $~Q,~$ then show that the locus of $~Q~$ is given by the equation :

$~x^{-2}+y^{-2}+z^{-2}=\frac{1}{ax}+\frac{1}{by}+\frac{1}{cz}.$

Solution.

Let $~Q \equiv (\alpha,\beta,\gamma).~$ So, $~L\equiv (\alpha,0,0),~~M \equiv (0,\beta,0)~$ and $~N\equiv (0,0,\gamma).$

The equation of any plane through the points $~L,M~$ and $~N~$ is given by $~~\frac x\alpha+\frac y\beta+\frac z\gamma=1 \longrightarrow(1)$

Let $~~P \equiv (x_1,y_1,z_1).~$ So, the direction ratios of $~\vec{OP}~$ are $~x_1-0,~y_1-0,~z_1-0~$ i.e., $~x_1,y_1,z_1.$

Now, $~\vec{OP}~$ is normal to the plane $~(1).$

$\therefore~ \frac{x_1}{\frac 1\alpha}=\frac{y_1}{\frac 1\beta}=\frac{z_1}{\frac 1\gamma}=k~~(\neq 0,\text{say})$

$\therefore~~x_1=\frac k\alpha,~~y_1=\frac k\beta,~~z_1=\frac k\gamma \longrightarrow(2)$

The point $~P~$ lies on the plane $~\frac xa+\frac yb+\frac zc=1.$

$\therefore~~ \frac{x_1}{a}+\frac{y_1}{b}+\frac{z_1}{c}=1 \\ \text{or,}~~ \frac{k}{a\alpha}+\frac{k}{b\beta}+\frac{k}{c\gamma}=1~~[\text{By (2)}] \\ \therefore~~ \frac 1k=\frac{1}{a\alpha}+\frac{1}{b\beta}+\frac{1}{c\gamma} \longrightarrow(3)$

Again, the point $~P~$ lies on the plane $~(1).$

$\therefore~~ \frac{x_1}{\alpha}+\frac{y_1}{\beta}+\frac{z_1}{\gamma}=1 \\ \text{or,}~~ \frac{k}{\alpha^2}+\frac{k}{\beta^2}+\frac{k}{\gamma^2}=1~~[\text{Using (2)}] \\ \text{or,}~~ \frac 1k=\frac{1}{\alpha^2}+\frac{1}{\beta^2}+\frac{1}{\gamma^2} \longrightarrow(4)$

Hence, from $(3)$ and $~(4)~$ we get,

$~\frac{1}{\alpha^2}+\frac{1}{\beta^2}+\frac{1}{\gamma^2}=\frac{1}{a\alpha}+\frac{1}{b\beta}+\frac{1}{c\gamma} \\ \text{or,}~~~ \alpha^{-2}+\beta^{-2}+\gamma^{-2}=\frac{1}{a\alpha}+\frac{1}{b\beta}+\frac{1}{c\gamma}.$

Hence, the locus of $~(\alpha,\beta,\gamma)~$ is

$~x^{-2}+y^{-2}+z^{-2}=\frac{1}{ax}+\frac{1}{by}+\frac{1}{cz}~~\text{(proved)}$

Post Views: 134

In the following article, we are going to discuss/solve few Short Answer Type Questions of S.N.Dey Mathematics-Class 12 of the chapter Plane. In the previous article , we have solved few Short Answer type Questions.

Leave a Comment Cancel reply